As with so many other things, there is no good theory of superconductivity. Physics now claims to know almost everything, but the number of good physical (mechanical) answers it has to questions is approaching zero.

Superconductivity is currently said to be explained by two theories: the Ginzburg-Landau theory (1950) and the Bardeen-Cooper-Schrieffer theory (1957). Notice first of all that one theory is 60 years old and the other is 53 years old. No theoretical progress in 53 years. It gets even worse when you look at the theories. GL theory is not a theory, it is just a lot of math. Even Wikipedia admits that GL is a mathematical model, not a physical theory, and that it "does not purport to explain the microscopic mechanisms giving rise to superconductivity." So we will pass it by without comment. No, I will pass it by with only this comment: this is the same Landau I critiqued in my paper on the Landau pole. He loves to bury problems under bad math. But since we are looking for an explanation, not math, we won't even pause to pull apart his math here.

BCS theory begins with this:

At sufficiently low temperatures, electrons near the Fermi surface become unstable against the formation of Cooper pairs.

What is a Fermi surface?

The Fermi surface is an abstract boundary useful for predicting the thermal, electrical, magnetic, and optical properties of metals, semimetals, and doped semiconductors.

What are Cooper pairs?

Cooper showed that an arbitrarily small attraction between electrons in a metal can cause a paired state of electrons to have a lower energy than the Fermi energy, which implies that the pair is bound. In conventional superconductors, this attraction is due to the electron–phonon interaction.

I hope you can see that we aren't in the presence of a mechanical theory here either. The Fermi surface is an abstract boundary, which means the theorists just made it up. We have no data confirming a Fermi surface, and we have no mechanical cause of the surface, so it is completely heuristic. The same can be said of Cooper pairs. Cooper proposes an arbitrarily small attraction, but provides no mechanical cause for it. It is a virtual attraction, in other words, a borrowing of attraction from the void. We see the state of the theory from this paragraph:

Although Cooper pairing is a quantum effect, the reason for the pairing can be seen from a simplified classical explanation. An electron in a metal normally behaves as a free particle. The electron is repelled from other electrons due to their negative charge, but it also attracts the positive ions that make up the rigid lattice of the metal. This attraction distorts the ion lattice, moving the ions slightly toward the electron, increasing the positive charge density of the lattice in the vicinity. This positive charge can attract other electrons. At long distances this attraction between electrons due to the displaced ions can overcome the electrons' repulsion due to their negative charge, and cause them to pair up. The rigorous quantum mechanical explanation shows that the effect is due to electron–phonon interactions.

That is not "a simplifed classical explanation," it is transparent sophistry. Here we have negative charge “increasing the positive charge density.” So we are being told that negative charge can INCREASE positive charge, which would be energy from nothing. The increased positive charge then attracts other electrons, so we have electrons attracting other electrons by this mechanism. They "pair up." Each sentence is a new miracle. Not one statement in that paragraph follows from the previous statement.

Good lord, how did we ever come to such a pass, that physicists can write and read drivel like that? We are told that the quantum mechanical explanation is rigorous, but if you believe that you aren't paying attention. How could the “rigorous” explanation be good when the simplified explanation is preposterous? Just as an example, we are told that the rigorous explanation depends on the phonon. What is a phonon? It is a quasiparticle. What is a quasiparticle?

It is one of the few known ways of simplifying the quantum mechanical many-body problem (and as such, it is applicable to any number of other many-body systems). The most well known quasiparticles are the so-called electron holes, which can be thought of as "missing electrons."

As always, the further you go, the worse it gets. It does not get more rigorous, it only gets more ridiculous. A phonon is a way to fill a hole, in other words. It is a thing that fits the hole in your theory, and then you call that thing a particle.

For more analysis of BCS theory, you may visit my newer paper on high-temperature superconduction, where I replace BCS and RVB theory with a fully mechanical model, including the complete molecular and nuclear diagram of a Copper Oxide ceramic.

But enough of that. If I wanted to be slapped in the face by a wet fish, I would have gone to the clown market. I want a physical answer to the question, "What causes superconductivity?" If the answer were really that difficult, I would understand all the misdirection. But it turns out the answer is fairly simple. All you need is the charge field. To get a charge field, all you do is let the photon that transmits charge be real instead of virtual. You let it have moving mass, radius, and spin. Since charge is real, it cannot be transmitted by virtual particles with no size or energy in the field. We don't have to propose a phonon to fill a hole in our theory. No, we just have to propose that the particles that our equations give us are real. I mean these old equations:

e = 1.602 x 10^-19 C

1C = 2 x 10^-7 kg/s (see the definition of the Ampere to find this number in the mainstream)

e = 3.204 x 10^-26 kg/s

Those equations tells us that charge has mass, and they tell us how much. The fundamental charge is that much mass per second, which I simply apply to the charge field and the photons that are in it. Charge is then the motion of these real photons, not some mystical attraction or repulsion of ions.

This solves the superconductivity problem because conductivity is defined as the ability of a substance to conduct charge. Normally, what is conducted is electricity, but at the fundamental level* electricity is charge conducted linearly by the nucleus, (while magnetism is charge conducted orthogonally). To see what I mean, we have to consult my diagram of Copper, which I have recently imported into this paper from my newer paper on Period 4.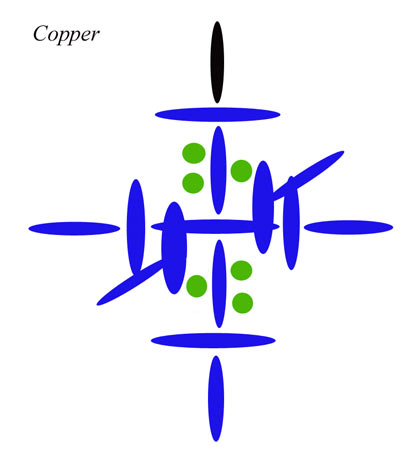



In short, Copper conducts well because it channels charge efficiently from south pole to north pole. All elements normally channel from pole to equator, and Copper still channels a large percentage that way; but Copper channels more from pole to pole than any other element except Silver. To understand exactly why, you will have to read that paper, but studying Copper helps us understand what conduction is as a matter of charge channeling. Once we understand that, we can comprehend what causes superconduction.

Under normal circumstances, a nucleus will channel from pole to equator due only to rules of angular momentum. Since the nucleus as a whole is roughly spherical, given any spin, there will be much more angular momentum at the equator. This will act to "pull" charge to the carousel level of the nucleus, where it will be emitted equatorially. Therefore, if we wish to maximize conduction from pole to pole, we have to minimize charge channeling at the equator. The primary way to do that is by stopping the spin of the nucleus. This is what happens with supercold superconduction. At very low temperatures, the carousel level stops spinning, and all charge channeling is then forced to happen along the poles. The spin stops because the ambient charge field has too little density to spin the nucleus. In that case, the only appreciable charge field is the field introduced externally (the field that is conducted). Since that field is unidirectional to start with, it cannot come at the nucleus from the side and therefore cannot spin it. The nucleus naturally aligns itself to the external and introduced charge stream, you see, so there is no way to spin the nucleus with it.

As I hope you can see, this immediately explains the Meissner Effect, and its relationship to superconductivity. The equatorial or carousel channel is orthogonal to the pole channel, and it is the equatorial channel that we normally call the internal magnetic field. If the nucleus stops spinning, this orthogonal channel is broken, and the magnetic field lines thereby disappear.

In this way, superconductivity can be explained with poolball mechanics. Current theory just doesn't have the right balls.

For more on superconductivity, especially high-temperature superconductivity, you may now consult my newer paper on solid light, Cooper pairs, BCS theory, and Copper Oxide ceramics, where I diagram a complete ceramic molecule consisting of Copper, Mercury, Barium and Calcium.

For even more on superconductivity, you may now consult my newer paper on superfluids.

*Electricity as we know it is the motion of ions, but it is the charge photons that drive the ions.

If this paper was useful to you in any way, please consider donating a dollar (or more) to the SAVE THE ARTISTS FOUNDATION. This will allow me to continue writing these "unpublishable" things. Don't be confused by paying Melisa Smith--that is just one of my many noms de plume. If you are a Paypal user, there is no fee; so it might be worth your while to become one. Otherwise they will rob us 33 cents for each transaction.