return to homepage HUBBLE REDSHIFTS AND THE
return to updates
COSMIC BACKGROUND RADIATION
the cosmological constant
by Miles Mathis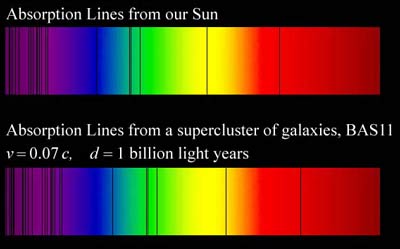
I address these two questions together for several reasons. One, they are the two main pillars of Big Bang theory. Two, they are both concerned with data coming in from great distances. Three, I will show that they are connected in ways not yet seen.
I want to preface this analysis with a statement of my allegiances, or rather my lack of them. I am neither a proponent of the Big Bang nor of the Steady-State. I am also not a proponent of tired light or any other theory. Although I admire the plasma physicists for their bucking of mainstream propaganda, I am not a plasma physicist or a colleague of Alfven or Lerner or any of the rest. I think Lerner's book The Big Bang Never Happened makes some interesting points, but I feel intuitively that his assurance is just as groundless as the assurance of the mainstream. The Big Bang may not have happened, but we are not in a position today to say what did happen to the universe, what is happening, or what will happen. Important data is coming in, but we only have a tiny fraction of the data we would need to make an educated guess. Our basic physics is also very full of very big holes, and that must limit our ability to make good guesses. This applies to plasma physics like the rest, and I have never found ambiplasma cosmologies to be at all convincing. Since there is no solid evidence of an anti-matter shortage, we don't require theories from either the mainstream or from the fringes to explain it.* I find cosmology in general to be a field of shocking presumption, and I will try not to add to it. I have no theory of the first few seconds, and no universal theories at all, except a few suggestions here and there. This is all to say that although I have blown very big holes in current theory, and will continue to do so, I am not claiming to have bolstered any alternative theory. We are much more in need of specific answers to specific questions, than we are in need of any more grand theories. My work is not the search for a final theory, it is the attempt to clear the board of final theories, so that we may once again look at the data with a fresh eye.
The first thing I want to draw your attention to is that the Cosmic Background Radiation (CBR) is currently said to be redshifted at a very high number for z. We know that the observed energy of this CBR is microwave, peaking at about 1.9mm. And we are told this observed wavelength has been redshifted 1,100 times. But if the CBR is a relic, as is currently supposed, I would say it could only be shifted the maximum amount. In other words, it should be shifted around 14 billion years worth, or a bit less. How much is that? According to current math and models, that should be a shift of around z=8. Applying that to our CBR photons with an average wavelength of 1.9mm, that would give us about 2 x 10-4m for the emitted wavelength.
But the current model finds z=1,100. Where did they get that? They got it from the relativistic redshift equations, which I have shown are completely compromised, not only by a false gamma, but also by many other mathematical errors. In my paper on the famous Pound-Rebka experiment, I have pulled apart the equations line by line, including the use of that equation by Feynman. I will not repeat the analysis here. Only with compromised math could contemporary physicists get such a ridiculously high number for z.
Using the much more logical number z=8, we find the original radiation had a wavelength of 2 x 10-4m. That number is of great interest to me, since I have shown that the charge field photon should have an average wavelength of about 1.6 x 10-4m. This number is easy to derive, so I will show you how to get it again. By pulling apart Newton's equation, I was able to show that it has always included the charge field. The constant G1 is simply the scaling constant between the charge field and the solo gravity field. G takes the size of one field down to the size of the other field, so that we can compare them directly, and put them both in the same equation. Because this is true, what G is really doing is scaling the atomic field down to the photon field. Charge is a force upon protons and electrons, mediated by photons, so we have to scale one to the other. Therefore, G is just the size differential between the proton and the photon. Yes, the photon is simply G times smaller than the proton. This gives the basic or average charge photon a mass equivalence of 1.1 x 10-37 kg. Using the common equation
λ = h/mc
We find a wavelength of about 2 x 10-5m.
The problem with that math is that G is a size differential, not a mass differential. We just treated it as a mass differential. Fortunately, we don't need to know the relative densities of the photon and proton to solve. We just let the mass stand for the size of the particle proper, and remember that the wavelength is determined by stacked spins. These spins give the particle more size, because they must obey gyroscopic exclusion rules. I have shown that, since most quanta have four spins, the outer spin is 8 times the radius. So to find the E/M wavelength differential from the mass differential, we just multiply by 8. That gives us a visible wavelength of 1.6 x 10-4m. That is still an estimate, since we still haven't included density, but it is a somewhat better estimate than our first number.**
That gives us a rough match of the wavelengths, near enough that I can propose that the cosmic background radiation is not a relic of the Big Bang, but simply the ambient charge field of the universe. I have been told that we have no evidence of my charge field at the macro-level, but I have shown that we actually have lots of evidence for it. A lot of evidence that is sitting right out in the open is evidence of the charge field, it has just so far been misapplied or misinterpreted.
As further indication that my math is preferable to the current math, remember that the current value of 1.9mm for the CBR was never really predicted, although we are told it was. If we study history without all the horn-tooting, we find the CBR as a relic of the Bang was never predicted at all. What was predicted was an ambient temperature of space, or of the universe, but that temperature was never assigned to the Bang until later.
As far back as 1896, Guillaume estimated the radiation of the stars to be about 5.6K. Eddington estimated about 3K for the same thing. In 1946, Gamow predicted 50K, but thought it was the actual temperature of interstellar space. Alpher and Herman, also speaking of a temperature of the universe, estimated 5K and then 28K a year later. In the 60's, Dicke estimated 40K, but still failed to link it to the Bang. In 65, Penzias and Wilson discovered the actual CBR, at about 3K. It wasn't until the 70's that the CBR was linked to the Big Bang, and amazingly no one (except Penzias and Wilson) won a prize for it, since no one had actually predicted that the CBR would be proof of the Bang. All the predictions had been about black body radiation or the temperature of space, not about a residue of the Bang. The residue theory was tacked on later, since without it Bang theory couldn't explain the CBR at all (and still can't).
Wiki tells us that “The cosmic microwave background is perhaps the main prediction of the Big Bang model,” but historically that isn't true. The Bang model used the CBR, but never predicted it. Wiki also tells us “Two of the greatest successes of the big bang theory are its prediction of its almost perfect black body spectrum and its detailed prediction of the anisotropies in the cosmic microwave background,” but that isn't true either. These authors are very loose in their use of the word “prediction.” What they mean is “fluffy explanation.” Big Bang theory has only followed data and co-opted it, it has never predicted it. Guth didn't propose inflation until 1980, and it is inflation that leads to the CBR. We even have a date for the CBR creation: year 379,000 (excuse me while I chuckle: why not 378,000 or 380,000?). Just ask yourself this: if Bang theory could explain the CBR without early thermal residue models, it wouldn't need an early thermal residue model, would it? Which means that the Bang model can explain the CBR only by speculating wildly about early times that we really have no data for one way or the other. To get to year 379,000 and the production of the CBR, we have to go from a singularity through periods of exponential inflation, symmetry breaking, and decoupling. So not only do we have no solid data, we have a string of illegal manipulations. I have shown in other places that all of these manipulations are illegal under any circumstances. There is no such thing6 as exponential inflation, symmetry breaking7 is a big fudge—requiring borrowing from the vacuum—and decoupling is just a word with no mechanics behind it. These theorists propose that photons and matter “decouple” by some sort of scattering, but they don't explain how the free photons got there in the first place without being emitted by baryons or electrons. They also don't explain how the baryons or electrons got there.
Bang theory is built upon a web of propaganda. Wiki tells us that “The discovery of the CMB in the mid-1960s curtailed interest in alternatives such as the steady state theory", but that isn't true, either. What curtailed interest in steady-state theories was a confluence of at least three main factors: 1) The ascendance of particle physics in the 1960's, fueled by accelerators and perceived advances in QCD, led to theory being dominated by particle physicists. These particle physicists were extremely revolutionary and ambitious, and they weren't interested in unsexy theories like steady-state theories or any other semi-classical theories. They knew that old science didn't sell. Bang theory was not created by astrophysicists. In the main, it was created by particle physicists. We are told that Bang theory evolved out of GR, but it mainly evolved out of QED and QCD. Just look above: symmetry breaking came out of electro-weak theory, since it is a gauge field manipulation. Symmetries are gauge symmetries. That is not GR, that is QCD. 2) The 60's were a time of revolution in general, and of sex. Novelty had been at a premium for the entire century, but in the 60's this trend peaked, in all fields, including art, cinema, music, and science. Everyone wanted excitement, not truth. They wanted titillation, not rigor. They wanted advancement at any cost. More than all that, they wanted to be “with it.” 3) The space race showed that science could demand and achieve huge budgets and that scientists could achieve fantastic levels of prestige. But they could achieve neither with old-fashioned science. Only with shocking new theories and hugely expensive proposals could they achieve their career goals. The sixties was the beginning of the rise of the propaganda state in the US, and the media circus. Science, like all else, was no longer a matter of sober research and rigorous work, it was a matter of public relations.
But back to the CBR. Notice that if we study the history closely, the explanation of the CBR by Bang theory is circular. The CBR is said to be one of the pillars of Bang theory, and the first and most convincing proof of it; but the Bang is used to prove the CBR and the CBR is used to prove the Bang. That is circular. As I have reminded you, the Bang cannot produce the CBR except by stacking almost all the illegal mathematical tricks QED and QCD have learned in the past century. All the “proof” of the CBR is in the first years of the Bang, hidden under piles of ill-defined or non-defined mathematical manipulations. This model of the early universe is not proof of anything, really, since it is no more than a bad trip on a chalkboard.
The CBR is now said to be definitive proof of the Bang, since steady-state models cannot account for the black-body spectrum we see. But this is not true. It was actually the Bang model that could not account for it: that is why they had to assign it to a residue of extraordinary early states. Because they didn't and don't know about the charge field, they couldn't and can't account for either the temperature or the curve by looking at normal matter in the present universe. The steady-state models before the 70's could not account for it either, it is true, since they didn't know about the charge field either; and no one has done much work on steady-state models since then. But my model can account for the spectrum quite easily. In the 60's the steady-state people didn't have any idea that the CBR might be the charge field of the universe. Right now, no one but me has any idea that the CBR is the charge field of the universe. But I have just shown that we can calculate (not estimate, not predict, but calculate) the peak wavelength of the CBR straight from Newton's equation and G. The standard model still cannot do that. It is not even close to doing that, since it still hasn't figured out that the charge field exists at the macrolevel.
My charge field follows a black body curve simply because the black body curve has always been a naïve representation of the unknown charge field. In nature, there is no such thing as a black body. Real objects that emit radiation are not black, and the more radiation they emit, the less black they are. Black body theory has always been a bit nebulous. It was never clear what was causing the spectrum. But the black body spectrum and curve follow the curve of the charge field, since that is what it is and always has been. Black body radiation is actually just the ambient or residual radiation of all matter, after you subtract away the radiation caused by all other mechanisms. For instance, a real body can be emitting for any number of reasons: it can be emitting for thermonuclear reasons, as with a star; it can be emitting because it is hotter than its surroundings, as with a fire; or it can be emitting just because it exists, as with everything else, no matter how small or cold. This last is black body radiation, and it is also the charge field. All matter that is not neutral emits simply because it is made up of spinning quanta, and this emission is what the black body curve follows. A real body acts more like a perfect black body when its density is near an optimum value, and that optimum value is determined by the spacing of the quanta. As I have mentioned elsewhere2, it is this density that determines a perfect black body, not the blackness or any other mysterious parameter.
For all these reasons and more, my math and postulates are much to be preferred to the current math and models, which have been pasted together over the decades from all sorts of half-baked speculations. I think anyone can see that it is better to derive the CBR wavelength from Newton's equation and G than to derive it by pushing cosmological models to match data.
Another big problem with the current inflationary model is that it breaks the rules of Relativity in spectacular fashion. We are told that in Special Relativity, objects cannot travel faster than c relative to one another, but in General Relativity they can. That is false, but even were it true, it isn't the problem here. The problem here is that the inflationary modelers have single discrete objects going way over c. In other words, they aren't exceeding c as matter of vector additions, they are exceeding c with single vectors. That is forbidden in both SR and GR, since in that case a single discrete object would be exceeding c. Claiming that inflation implies one object going over c relative to another object is just misdirection. That isn't what is happening. What is happening in inflation is that primordial objects are going way over c in their own coordinate systems. That simply isn't allowed in either SR or GR, because it would take the mass to infinity. We are told that in the inflationary epoch, the universe increased in volume by 1078 in 10-32 seconds. Assuming the universe then was already at least one meter in size, that implies an average velocity of at least 10108c. To avoid exceeding c, the final size of the universe after inflation would have to be about 10-25 meters, or roughly the size of one of my charge photons.
To answer this problem, they will say that inflation is an expansion of space, not a velocity of particles, but that is just a hedge. If the universe were expanding at the rate they say, then everything in that universe would be expanding at at least the same rate. Unless they are proposing early particles are moving backwards to expansion.
Which brings us to another problem no one ever seems to see. In the primordial universe, all matter should be in its most primordial state, right? It can't yet have formed more complex particles, not even electrons or protons. Which means the first particles are probably photons, or particles very much like photons. Remember, photons go c precisely because they are so small. The zero or very low mass is fundamentally connected to the speed c, although they usually don't admit that or tell you that. Well, that is a problem because then what they have going more than a googol times c is photons. Yes, they have light going 10108c. How can they say that doesn't contradict Relativity? It contradicts the first postulate of both SR and GR.
Notice they misdirect you in numerous ways. First they tell you c is not a limit in GR. False. Then they tell you c is not a limit in GR when we do vector additions: when we are comparing one velocity to another. In most cases, that is also false. But even when it is true, it doesn't apply to the problem at hand. Inflation isn't a vector addition, it is a vector. The speed of a single photon is not a vector addition, it is a vector. During inflation, photons would be traveling googol c. They are trying to apply vectors to space, but that is mathematically impossible. You can't apply vectors to spaces, you can only apply them to objects. They are assigning numbers to NOTHING.
To see the mess this causes, just apply the idea to photons NOW. They tell us that space is expanding now, at an accelerating rate. Well, even a low acceleration will surpass c in a relatively short time, so photons now must be going way over c. You should ask them to tell you how much. Are they going a googol c now? If they are, what does that do to Relativity? What does it do to mass increase? You see, they propose these ridiculous things and then limit their application. Every problem has its own rules and its own ad hoc solutions. Don't apply the logic of inflation to black holes, or you will be disappointed. Don't apply the logic of quark theory to celestial mechanics, or you will be disappointed. Study every problem with blinders on, and you will be thrilled.
Now we look closely at Hubble redshifts. Notice that my analysis does not fully contradict the Big Bang or expansion (yet). In fact, I have used Hubble redshifts to bring the numbers together, so most would say my proposal depends on current theory. Yes, it does depend on redshifts, that much is clear. But my paper is only half done.
We are told that no other theory can explain these redshifts. And in the past decade or two, we have had another broad finding to explain: increasing redshifts. That is to say, not only do the redshifts increase with distance, they also appear to be increasing with time. This is now generally interpreted to mean the expansion is increasing, and to mean that the universe is open.
Is it true that there is no other way to explain both these phenomena? Let us consider two broad facts, facts that have never been studied closely in this context, or studied as a pair. One, the Earth is on an outer arm of a large spiral galaxy. This means that the Earth and everything in its vicinity is moving in a large arc through the universe. Now, the light that is reaching the Earth from beyond the galaxy may also be moving in a large arc, due to the curvature of the entire universe, but the curvature of this arc must be much smaller than the arc of the Earth. The universe cannot be curving at the same rate as a single galaxy. Some will answer that light IS bent by the galaxy, since light is bent by all mass, but, again, the light cannot be bent as much as the Earth's motion is bent, since the light is moving so much faster (1,400 times faster). The Earth's bend could be seen just by looking at the galaxy from a distance, while the light's bend would not be obvious at all from the same vantage. No matter how you look at it, the Earth is moving in a tighter curve than the light reaching it from all sides.
Two, this great arc of the solar system as it revolves around the galactic center is not circular, but elliptical. This means that the curvature changes slowly over time. The solar apex, which measures the current direction of the Sun and its system relative to the galactic center, is currently at 60 degrees. If the current orbit were perfectly circular, then that angle would be 90 degrees. From this we can conclude that the Sun and its system are currently experiencing a greater arc than normal or average, and that therefore we could say the curvature is increasing. In other words, the Sun must be approaching one of the “ends” of its ellipse, the galactic equivalent of either perigee or apogee.
Now, these two facts taken separately don't point to much. But together they may suggest another cause of Hubble redshifts.
The first thing to notice in this regard is that the relationship of the curve of the Earth to the lesser curve of light is absolute: it doesn't change with regard to direction. The galactic motion isn't curving one way to light coming from one direction and curving the opposite way to light coming from another direction. The difference in curvature is an absolute. For example, draw two circles, one bigger than the other. The bigger one will have less curvature at all points, and this curve relationship is blind to direction. A curvature difference is an absolute. If this isn't clear, look at the cause of the Earth's curve, instead of the curve. The curve is caused by gravity. Well, light coming from all directions experiences the gravity of the Milky Way in the same way. Gravity doesn't curve in for light coming from the east and curve out for light coming from the west. It always curves in.
The second thing to notice is that we have a magnifying of this effect. The Earth isn't just curving with regard to the galactic center, it is also curving with regard to the Sun. The Earth is in two gravitational curves simultaneously, and it is curve is much greater than the distant light it receives. This may be important, since we can say the same thing about all of our telescopes to date. Even the ones that have left the Earth's surface have still been orbiting the Sun with us.
The third thing to notice is that we may have a second magnifying effect. The Earth is also rotating on its axis, so we have a tight curve about the center of the Earth. That makes a third curve that the light itself is not experiencing. The Hubble Space telescope would also be expected to experience this second magnifying effect, in fact even more, since it is orbiting once every 95 minutes.
Now I just have to prove that this difference in curvature would cause a redshift. This isn't hard to do, since gravitational fields are already known to cause redshifts, and this is admitted by the standard model. Curvature is the sign of gravity, so all straighter lines must be “less gravitational”, in at least one sense. A critic will say, “If the Earth is in two or three curved fields, by your definition, then isn't it downhill in two or three ways? And, if so, shouldn't it actually be blue-shifted two or three times? Gravitational redshifts require that the observer be uphill, as with the Earth observing a star like Sirius. This also applies to your crazy expansion theory, since if the Earth is expanding, it should be moving toward all incoming light. That is also a blue-shift!” I admit that is the knee-jerk analysis here, and I have made it look pretty damning on purpose. But it can be answered.
The answer is that gravitational shifts like the ones my critic is talking about are very small. That is why they have been difficult to confirm. If they were as large as the Hubble shifts we are trying to explain here, they would have been easy to confirm. We wouldn't have to study Sirius or fudge experiments in short towers at Harvard5, we could get confirmation straight from the Sun. The Sun has a huge gravity field, and we should have found easy confirmation there. This is all to say that I am not proposing that sort of uphill/downhill small effect. It would not help me here for many reasons: 1) it is too small, 2) it is blue instead of red. No, I am going outside of and beyond current theory once again, although as usual I will not completely contradict current theory. I have never wished to contradict either Relativity or gravity: I only wish to correct and extend them. In this case, I don't need to address Relativity, since gravity was already a curve before Einstein, and the main curve is all I need here.
What I mean by that is that gravity was always an acceleration, and any acceleration can be written or expressed as a curve. Just consider a Cartesian graph, where all accelerations are curves. A Cartesian graph is not relativistic. So we could have expressed Newton's gravity as a curved field if we had wanted to. Einstein's field is actually two curves stacked, but Newton's field was already the main curve, and that is what I am unwinding here, in a novel way.
To understand what I am about to say, you have to cleanse your palate of all previous causes of redshift. Clear your mind of Doppler effects and gravitational redshifts. Clear your mind of Relativity. All you have to accept is that we have real curves here, caused by orbiting. It turns out that any curve will measure any straighter line to be redshifted, supposing that line is a “line” of light or E/M radiation. The rather simple reason for this is due to the operation of measurement, and all we have to do is look at how we see distant objects using light. The important fact is that we do not measure at an instant. When we collect light from a distant object in a telescope, we take some sort of extended reading. It will usually not be too extended, since we want to avoid blurring, but we don't just collect one dt of light or something and do our calculations from that. No, we have an exposure time of some seconds or minutes, and that gives us our “observed wavelength.” Now, if we have our telescope on a planet that is rotating once every day, or on a low-orbit satellite that is orbiting once every 95 minutes, and that planet and satellite are orbiting a star which is orbiting a galactic center, that minute of light collection will be a curved minute. To the light, it will be non-negligibly curved, even over a short period of time. Remember, the light is moving extremely fast, so one minute allows for a lot of change in light. Light can change 18 million kilometers in one minute, so one minute is never negligible to light. The light we take in at the beginning of our minute is not strictly equivalent to the light we take in at the end of our minute, though our telescope is turning very slowly to accommodate the source. The curvature is registered in our collection, and it is registered as a redshift. It is registered as a redshift because the curve always creates some sideways motion to the light during the collection of the light.
Look at it this way. If I am moving in a curve relative to you, that just means that you can't measure me with only one dimension. You can't measure me using only x, you need x and y. Over any interval, I will be moving some amount sideways to you. If we reverse the measurement, this is also true. If I am moving in a curve relative to you, then you will be moving in a curve relative to me. I will define myself as motionless, or at least as uncurved, and I will apply the curvature to you. Therefore, I will have to measure you using both x and y. I cannot measure your motion with one dimension only. But of course this gives your motion a hypotenuse over every interval, and I have to triangulate to measure you.
Now, if we apply this pretty simple logic to a collection of light, it implies a blue shift only if I measure the same photon twice. For instance, if we could set up two detectors, one right behind the other, and the first detector could somehow make a detection without stopping the photon, then the two detectors would show a blueshift of that one photon as it traveled down. That would be the gravitational blueshift. But that is not what is happening with a real telescope, is it? It doesn't measure a shift on one photon, or even the shift of a set of photons, since it doesn't measure the same photons twice. No, it measures the first photons against the last photons. Since there has been a curve between the first photons and the last photons, there has been a sideways motion in that time, and it will look like the photons had to travel that hypotenuse. They will be stretched out by that curvature during the time of collection, and that stretching will be read as a redshift.
My critic will shout, “You don't have nearly enough motion or curvature to show the kind of redshifts we see, even if we give you an hour or a hundred hours! Those hypotenuses are tiny, and we can ignore them.” Can we? I remind my critic that the solar system is moving 220km/s in its orbit around the galactic center, and that the Earth is moving another 30km/s in orbit around the Sun, and that the Earth is moving another .5km/s around its own center. Those are some pretty spectacular speeds, especially the first one, which is c/1,400. Using just that speed, we can create an extra distance in one minute of 13,200km. That is not the kind of curvature you can ignore.
My critic will now say, “OK, but your analysis implies that the greater exposure time we have, the greater redshift we should find.” No, it doesn't. To find those hypotenuses, we compare the sideways motion due to curvature during the time of collection or exposure to the distance light travels in that same time. If we take a smaller time of exposure, we also have a smaller distance traveled by light in that time, so when we calculate a percentage change, it doesn't matter what time we actually expose. What matters is the curvature of the observer relative to the curvature of the light.
I have shown that curvature can cause a redshift by creating sideways motion relative to light. But can I show that light from more distant sources would be shifted more? Yes. Since we are comparing curvatures, all I have to do is show that more distant light is curved less. This is easy to do, since, in general, the universe is curved more at smaller scales than at larger scales. General Relativity shows us that the universe as a whole is curved, but the universe is not as curved as a galaxy, and a galaxy is not as curved as a star. The curvature decreases at larger scales, by definition. If the curvature increased for larger scales, the larger scales could not be larger, could they? They would be pulled back on themselves, and would be smaller. Therefore, light traveling longer distances can be considered to be “larger-scale” light. Its total bend may be quite large, but its bend per unit length is less than nearer light, simply because it got here from so far away. If it had the same bend per distance traveled as nearer light, it could not have gotten here from there. More distant light is bent less by a tautology, since to get here from there, it had to travel a straighter path. The universe is curved less at larger scales, and it is the universe that determines the path of the light. Light diverted by smaller scale curvatures could not have gotten here from so far away.
Since more distant light is curved less, the difference between its own curvature and our curvature will be greater. Therefore our sideways motion relative to it will be greater, and the redshift will be greater.
In a similar way, we can explain the increase in redshift discovered by the Hubble telescope. I showed above that the Sun and its system are currently in a phase of increased curvature, due simply to the elliptical nature of the galactic orbit. This means that this acceleration of the redshift is temporary. As soon as the Sun passes whichever end of the ellipse it is approaching, this acceleration of the cosmic redshift will switch to a deceleration.
I have addressed the main points of my theory, and will now try to reintegrate the CBR into what I have discovered about redshifts. If redshifts are caused by curvature, then we can explain the CBR with curvature instead of expansion. Everything I have said about other light applies equally to the CBR. We only have to keep our proposal that the CBR is, on average, coming from a great distance. Since I have proposed that it is the universal background charge field, rather than any local charge field, this is not difficult to do. As the ambient charge field of the universe, its average distance would be very large. In fact, using my equations above, we could even take the average out a bit. To match my charge field calculations more closely, we need the number for z to be about 11, which is well beyond the 14 billion year limit.
You will say I seem to have a strange mixture of old theory and new here, since values of z have to be read in terms of Doppler redshifts, not curvature redshifts. True, but I assume curvature would have to follow distance in much the same way that expansion did, since I am not overthrowing GR. I don't believe more distant objects are moving away from us, but I do believe the light they send us is curving less, and that belief is due to a general agreement with GR. In other words, Doppler changes are caused by distance, and so is my new curvature change. Because I don't disagree with the way the Doppler changes are calculated, I simply let my curves change at the same rate as the redshift, and in this way I have a first estimate of the distance.
Of course, I am not predicting that the universe is big enough to hold a shift of z=11. This paper is not about that. This paper is about the CBR and the cause of Hubble shifts. I have a lot of work left to do before I can begin to correct current theory beyond that. For one thing, my pulling apart of Newton's and Coulomb's equations, and finding the charge field within, will vastly change the mass we have to work with in the known universe. You can see without reading beyond this paper that I have given the photon a mass equivalence, and that requires we start over from the beginning in almost every subfield of physics. Obviously, I cannot and could not estimate a size of the universe without redoing hundreds or thousands of basic equations, and I have not done that. I take these problems as I find them.
Before I conclude, I will mention one more thing that ties in here. I have shown in a series of other papers that celestial mechanics3 lacks a balancing mechanism. Specifically, orbits show a degree of float that is completely unexplained by current theory, either Kepler's, Newton's, or Einstein's. In opening up Newton's gravitational equation, I have found the E/M contained within, signaled by G. What this has allowed me to do is to show very simply that the gravity field is and always has been two fields masquerading as one. Of course, this means that Einstein's field equations are also unified field equations, expressing both E/M and gravity at the same time. This makes every orbit a balancing of three motions, not two, which allows for stability and correction. It also explains resonances, torques, and many other things, in strictly mechanical ways. It allows us to put a ground under Laplace's equations8. But I have recently discovered that this same finding solves the problem of the cosmological constant, since the instability of the orbit and the instability of Einstein's field equations is caused by the same thing, and solved by the same thing. The reason Einstein's solutions were unstable is that he was unaware of the E/M field inside his equations. He was unaware that Newton's field, which he was extending and correcting, was already two fields. So when physicists—and Einstein himself—discovered that his equations were unstable, what they were really discovering is that the orbit is unstable. They were uncovering a problem that had been hidden for centuries. Kepler's9 and Newton's orbits are unstable, and have been from the beginning. But everyone had forgotten that, if they had ever realized in the first place. Newton's own field equations were not stable, because any bad differential would doom them. Orbits could be summed, to show a circuit, but the differentials themselves could never be explained. Any dt of imbalance should doom an orbit, according the historical and present equations, but orbits correct themselves somehow. Underneath the differential equations, the “innate velocity” of the orbiter makes corrections upon itself, though this is physically impossible in gravity-only theory.
Now, when Einstein added time differentials to Newton, and used tensors to express curvature, all this was buried a second time. Everyone thought that Einstein's equations had developed a new instability, when all they were doing is expressing an old instability in a new way. Einstein tried to correct this old instability by creating a constant that would force a new stability on the equations by fiat, but it turned out that data was even then coming in that showed instability. Hubble's data appeared to show an expansion, so the constant was changed to match this expansion. It has been changed several times since then to match newer values of expansion.
However, all this will turn out to be a historical dead end, since I can correct Einstein's equations just like I did the orbit and the ellipse and Newton's equation. Once we realize that Einstein's field equations contain the E/M field, we can show how the stability is created by the mechanics of the two fields. I have showed that the orbit is really stable for a reason, since the E/M field creates that stability by responding to changes in the gravity field or to changes in position. And when we open up Einstein's field equations and see the E/M field there, we get the same stability. Newton's and Kepler's equations only seemed to be unstable, because they had been mechanically undefined, or under-defined. In the same way, Einstein's field equations only seem to be unstable, because they have not been recognized as unified field equations10. Yes, Einstein never realized that his equations were already unified. The solution was hiding in plain sight.
This means that the cosmological constant is just a fudge, no matter what its value is. Einstein's equations don't require any constant. They require a subtle reworking and re-defining, so that we see that some of the rates of change of some of the tensors are defined by the E/M field, working in tandem with the gravity field. Once we see this, and express this correctly, the equations will be self-correcting. It is the charge field that allows for this correction and unification of both Newton's and Einstein's equations.
I have already shown in other papers11 the full mathematical correction of the overall strength of Einstein's field equations, proving with simple math and postulates a general 4% error in the field of the Sun. This correction fully and immediately explains the current Saturn anomaly11, as well as the older Pioneer anomalies. But Einstein's field equations also require a cracking open, to re-assign certain tensors. Those who are interested in keeping the tensor calculus and the curved field will have to do that themselves, since I am interested in neither. I have shown that we can express GR with a Euclidean field and simple math, and I prefer to show the charge field with that math. I have already begun to do that in several papers12, where I “relativize” Newton's unified field equation just by adding time differentials to the algebra directly. But there is much more to do.
I mention this because the Big Bang and expansion have been closely tied to the field equations and the value of the cosmological constant from the beginning. If I have thrown out expansion and the current explanation of the CBR, I also have to address the constant. I want to make it clear to a reader of this paper that I have done that. The cosmological constant is just another in a long line of ghosts, ghosts that I have dissolved completely. There is no constant and no field instability, since the field equations, like the orbit, can be fully stabilized in a natural way, by the charge field. We don't fill Einstein's hole with a constant, we fill it with the charge field.
If you have penetrated any of that, you will understand why I am with neither the mainstream nor the plasma physicists. The mainstream has done too little with the charge field, and the plasma physicists have attempted to do too much with it (while not even being able to define it or place it in field equations). Since I have pulled the foundational E/M field or charge field right out of Newton's equation, I can confirm the unified field, but I cannot confirm either the primacy of gravity or the primacy of E/M. I have shown that neither are primary. Both are fundamental and both are ubiquitous. I agree with the plasma physicists that E/M must play a much greater role in the universe, but I also agree with the mainstream physicists who demand that E/M has a diminishing role at larger scales. They are both right in that. The charge field is everywhere, and must be factored in to all equations, including field equations. The photon must be given mass, and must be a larger player in all equations. But at the same time, the E/M field does diminish in importance at larger scales, since it drops off more quickly than gravity. As a real emission field (as opposed to a virtual field) emitted by spheres, it must dissipate fairly rapidly. It's influence in the Solar System is much greater than thought, simply because the emission is much greater than thought, and this probably applies to galaxies as well. But I tend to agree with the mainstream that outside galaxies, and between galaxies, the E/M field influence may be slight. Just look at the CBR. Even if the CBR is what I say it is, a 3K influence between galaxies is not much. Then again, we have probably miscalculated the gravity influence between galaxies, as we have miscalculated everything else. So we have yet to accurately measure one field against another, at any level.
Conclusion: Since redshifts may be explained by curvature, we don't necessarily need expansion to explain them. And since the CBR can be explained as the ambient charge field, we don't need to explain it as a residue of the Bang. However, as I said in my preface, I don't see this as any sort of proof against a Bang. I have disproved a hatful of human theories and a cartload of human conjecture, but I have proved or disproved nothing about the universe. The only thing I have proved beyond a doubt is that our current explanations are very incomplete, and that the mainstream jumped the gun in assigning Hubble redshifts to distance alone. As another example, I have recently shown5 that the gravitational blueshift equations are completely compromised and incomplete. I have reworked them and extended them, but they are still incomplete. The light we receive in a telescope from beyond the galaxy must be blueshifted at least three times, by the Earth's field, the Sun's, and the galaxy's.
I didn't offer any equations here to show how much of the redshift we see is due to curvature. And, as I just said, we don't know the total blueshift of the incoming light either. Beyond that, there may be other causes of shift that we haven't even begun to recognize. We don't have a proper tally of the shifts we know about, much less the shifts we don't know about. In my opinion, it would be best to admit this, and shelve the cosmologies for later. Since I have shown that both GR and QED are filled with major holes, and demonstrably wrong in important ways, I have no faith in Bang models that claim to know what was happening 13 or 14 billion years ago. A standard model that cannot explain gravity or orbits3 in its own solar system can hardly explain the genesis of the universe. A standard model that is 4% wrong about the Sun's gravity field cannot be close to correct about the gravity field of the universe. A standard model that cannot assign charge4 mechanically (it still uses little plus and minus signs!) cannot assign an age to the universe, or a future.
**This estimate can also be achieved by cubing the Dalton, 18213.
1http://milesmathis.com/g.html
2http://milesmathis.com/stefan.html
3http://milesmathis.com/cm.html
4http://milesmathis.com/charge.html
5http://milesmathis.com/pound.html
6http://milesmathis.com/inflat.html
7http://milesmathis.com/weak2.html
8http://milesmathis.com/laplace.html
9http://milesmathis.com/ellip.html
10http://milesmathis.com/uft2.html
11http://milesmathis.com/sat2.html
12http://milesmathis.com/uft.html
If this paper was useful to you in any way, please consider donating a dollar (or more) to the SAVE THE ARTISTS FOUNDATION. This will allow me to continue writing these "unpublishable" things. Don't be confused by paying Melisa Smith--that is just one of my many noms de plume. If you are a Paypal user, there is no fee; so it might be worth your while to become one. Otherwise they will rob us 33 cents for each transaction.